Which Are Crucial Points?
Critical points are crucial in calculus to find minimum and maximum values of charts.
Let’s say you purchased a new puppy, and went down to the local hardware shop and purchased a brand new fence for your lawn, but alas it will not come built. Obviously, this usually means that you get to fencing in whatever size lot you want with limitations of how much fence you have. Wouldn’t you need to maximize the total amount of space your dog had to operate? Critical points can tell you the specific measurements of your fenced-in yard that will provide you the utmost area!
Crucial points in calculus have other applications, too. For instance, they could let you know the lowest or lowest point of a suspension bridge (assuming you can plot the bridge on a coordinate plane). First, let us formally define what they are.
Definition of a Critical Point
Permit f be described at b. In the case of f(b) = 0 or if ‘f’ is not differentiable at b, then b is a critical amount of f. If this critical number has a corresponding y worth on the function f, then a critical point is present at (b, y).
Just what does this mean? Well, f merely reflects a purpose, and b signifies the stage or the amount we are looking for. The next portion of the definition informs us that we can set the derivative of the function equal to zero and solve for x to acquire the critical number! The third part claims that crucial numbers may also show up at values in which the derivative does not exist. We’ll look at an example of this a bit later. Lastly, if the important number could be plugged back to the original purpose, the x and y values we get will be our crucial points. Related – Difference Quotient | Formula, Calculator, Examples
Finding Critical Points
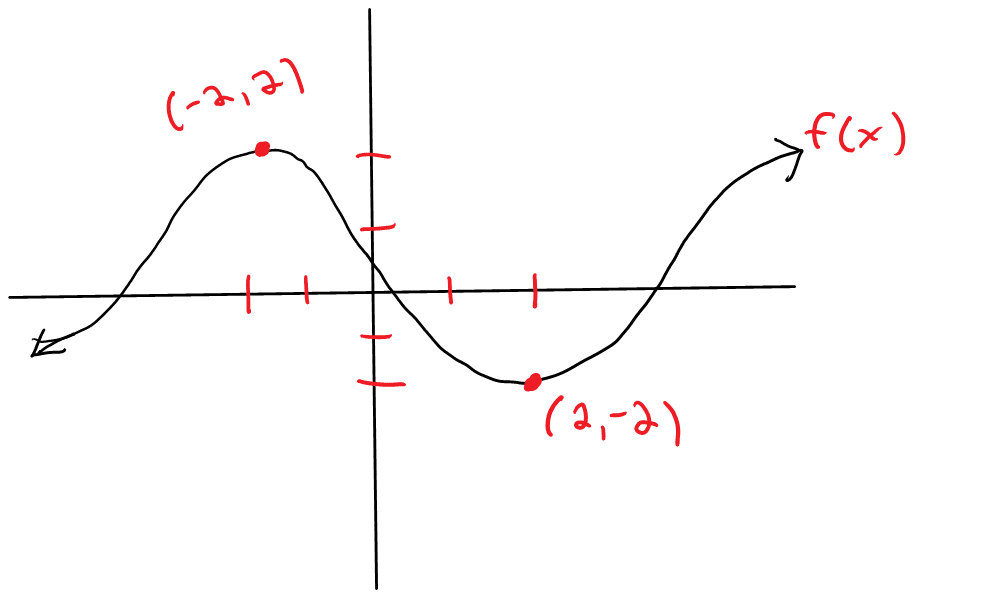
Now we’re going to take a look at a chart, point out some essential points, and try to find why we set the derivative equal to zero.
The red dots in the chart represent the critical points of that particular function, f(x). It’s here where you should Begin asking yourself a few questions:
Is there something similar about the locations of both essential points? You ought to start looking for visual similarities.
How does this compare to the definition from above?
Should you understand the answers to those two questions, then you can understand the way we find critical points.
Notice how both essential points often appear on a hump or curve of the graph. More specifically, they are at the very top or bottom of these humps. It’s why they are so critical!
To fully grasp the number one relates to the defection of a critical stage, we must recall what a derivative tells us. The derivative of a function, f(x), provides us a new function f(x) that represents the slopes of the tangent lines in every specific stage in f(x). So why do we set those derivatives equal to 0 to find critical points? Have a peek at the next graph that reveals different tangent lines into f(x).
The green tangent lines operate through our crucial points. What is the difference between these and the blue ones? For one thing, they have the same slope, whereas the blue tangent lines all have distinct slopes. For another thing, that incline is obviously one very specific number. Who recalls the slope of a horizontal line? That’s perfect! The slope of each tangent line that passes through a vital point is always 0!
More For You: