Difference Quotient is used to compute the slope of the secant line between two points on the graph of a function, f. Only to review, a purpose would be a line or curve which has only one y value for each x value. The difference quotient is a measure of the average rate of change of this function within a period (in this case, an interval of length h). The limit of the difference quotient i.e.(the derivative) is thus the instantaneous rate of change.
In calculus, the difference quotient is the formulation used for locating the derivative that’s the difference quotient between two points that are as close as possible which provides the rate of change of a function at one point. The difference quotient was formulated by Isaac Newton.
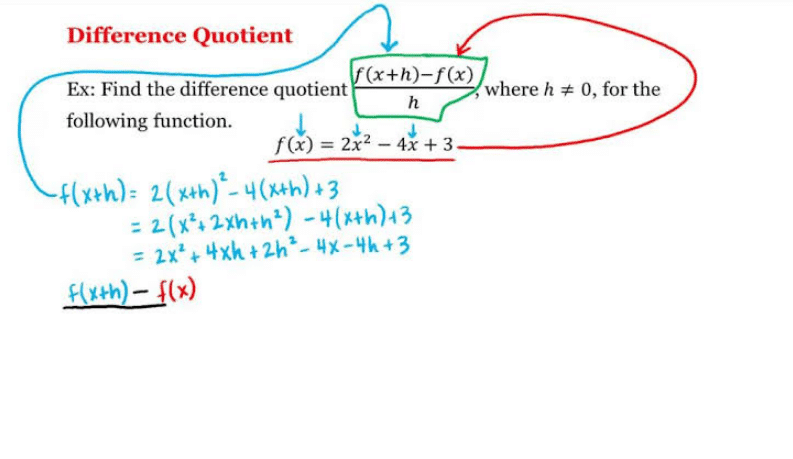
This formula computes the slope of this secant line through two points on the graph of f. These are the points together with x-coordinates x and x + h. The difference quotient is used in the definition of the derivative. First, plug (x + h) into your function where you find an x-ray. When you find f (x + h), you can plug your values into the difference quotient formula and simplify from that point. In the next step, you use the subtraction signal to eliminate the parentheses and simplify the difference quotient.
From the proper definition of this difference quotient, you will note that the slope we are calculating is to get the secant line. A secant line is just any line that moves between two points on a curve. We label both of these points as x and (x +h) on our x-axis. As we are working with a purpose, these points are tagged as f (x) and f (x + h) on our y-axis, respectively.
Difference Quotient Formula
The measures we take to locate the difference quotient are as follows:
Plug x + h to the function f and reevaluate to find f(x + h).
Now you have f(x + h), find f(x + h) – f(x) by plugging in f(x + h) and f(x) and simplifying.
Plug your result from step two into the numerator in the difference quotient and simplify it.
The name of this expression stems from the fact that it is the quotient of the difference of the value of the function from the difference of the corresponding values of its argument (the latter is (x+h)-x=h in this instance). Related – How to Calculate Molar Mass
Symmetric Difference Quotients
In mathematics, the difference quotient is formulations that give approximations of the derivative of a function. There are some different quotients, and those are the one-sided difference quotients and the symmetric difference quotient. They’re all related, and one gives a much better approximation than others due to this relationship.
The expression under the limit is sometimes called the symmetric difference quotient. A function is said to be symmetrically differentiable at some point x if its symmetric derivative exists at that point.
If a function is differentiable (in the usual sense) at some time, then it is also symmetrically differentiable, however, the converse is not true. , which is not differentiable at x = 0, but is symmetrically differentiable here with symmetric derivative 0. For differentiable functions, the symmetric difference quotient will provide a superior numerical approximation of the derivative than the usual difference quotient.
The symmetric derivative at a specific point equals the arithmetic mean of the left and right derivatives at the point if the latter both exist.
Neither Rolle’s theorem nor the mean value theorem hold for the symmetric derivative; some similar but weaker statements have been demonstrated.
More For You: